Someone recently sent me a link to a really terrific crank. This guy really takes the cake. Seriously, no joke, this guy is the most grandiose crank that I’ve ever seen, and I doubt that it’s possible to top him. He claims, among other things, to have:
- Demonstrated that every mathematician since (and including) Euclid was wrong;
- Corrected the problems with relativity;
- Turned relativity into a unification theory by proving that magnetism is part of the relativistic gravitational field;
- Shown that all of gravitational/orbital dynamics is completely, utterly wrong; and, last but not least:
- proved that the one true correct value of
is exactly 4.
I’m going to focus on the last one – because it’s the simplest illustration of both his own comical insanity, of of the fundamental error underlying all of his rubbish.
Let’s start at the beginning, with his introduction:
Historically, pi is the numerical relationship between the diameter and circumference of a circle. It is a geometric constant. What do we mean by geometric? Operationally, geometry is the study of drawn figures. The ancients actually drew their figures on paper (and some of us still do). All the concepts of geometry applied to these figures. A line was a drawn line. A circle was a drawn circle. Of course geometry soon invented some other postulates to help with the mathematics. A point was defined as having no extension, a line was defined as having no width, and so on. But the equations were still understood to apply to the figures. Geometry was always only partially abstract.
In this context, pi was assumed to be a dimensionless constant. It transformed one length to another. This is clear from the basic equation:
.
You can see that pi takes us from one length to another and therefore we must assume it is dimensionless.
What I will show in this paper is that this assumption is false. I will show that pi is not dimensionless. It is not dimensionless for the basic reason that the circumference is not a length. Nor is it a distance.
Quite a lot of crankery, right there. Some of it actually makes a bit more sense that it appears at first glance. I’m not saying that it actually make sense, but that given his rather peculiar definitions (which he discusses elsewhere), it’s not quite a bogglingly nonsensical as it appears.
One of his fundamental ideas is that Euclid got the concept of a point wrong. According to Mathis, there are two kinds of points: drawn points, and mathematical points. And you can only meaningfully apply numbers to drawn points; any attempt to assign a number to a mathematical point is completely erroneous. As an implication of this, all numbers must, inevitably, have units. There is, in Mathis’s world, no such thing as a dimensionless number: a dimensionless number is an abstract point, which can’t have a number assigned to it.
Back to his words, as he tries to explain some of this in the context of geometry:
Geometry dismisses time as a consideration. Geometry is understood to be taking place at a sort of imaginary instant. For instance, when we are given or shown a radius, we do not consider that it took some time to draw that radius. We do not ask if the radius was drawn at a constant velocity or if the pencil was accelerating when it was drawn. We don’t ask because we really don’t care. It doesn’t seem pertinent. It seems quite intuitive to just postulate a radius, draw it, and then begin asking questions after that.
It turns out that this nonchalance is a mistake. It is a mistake because by ignoring time we have ignored many important subtleties of the problem of circular motion and of circle geometry.
It’s hard to overstate just how completely and utterly wrong this is.
The fundamental goal of mathematics is abstraction: that is, it’s about taking something that you want to study, and focusing narrowly on that, discarding anything that isn’t essential to understand it. Euclidean geometry is concerned with shapes; it doesn’t matter how they were drawn, or whether they were drawn at all.
But Mathis is absolutely obsessed with idea that drawing something is absolutely critically important. A drawn point is meaningful; an abstract mathematical point is not. A drawn line is different from an abstract mathematical line. And, obviously, a drawn circle is fundamentally different from an abstract circle. Per his reasoning, an abstract circle isn’t particularly meaningful. You can’t (according to him) do something like compute the ratio of the circumference to the diameter in an abstract circle – because that would be applying numbers to something formed of abstract points.
Let’s see a bit more of where he goes with this:
As a simple example of this, when we draw a circle on a Cartesian graph, e make an entirely different set of assumptions than the ones above, although few have seemed to notice this. You would think you could draw a Cartesian graph anywhere you wanted and it wouldn’t make any theoretical difference to the geometry. You could draw a graph on the wall, on the floor, on any flat surface. You would think all you are doing is making things a bit easier on yourself as an artist and a geometer. Just as the old artists would square off their paper in order to make drawing a head easier, a geometer squares off a section of the world in order to create a tidy little sub-world where things can be put in order.
But all this is completely false. Drawing the graph changes everything. If you draw a circle without a graph, then you can say to yourself that the line (that is now the circumference of the circle) is a length. As a length, it can have only one dimension. A length is a one-dimensional variable, right? Perhaps you can see where I am going with this, and you say, “Wait, a circle curves, so we must have two dimensions, at least. We must have an x and a y dimension.” Yes, at the least we must have that. You saw this because you began to think in terms of the Cartesian graph and you could see in your head that the curve implied both x and y dimensions. Very good. But you are not halfway there yet. Take the circle and actually put it into a Cartesian graph. What you find is that the curve is now an acceleration. In fact, any curve is an acceleration in a two-dimensional graph. We all learned this in high school, although I don’t think it sunk far in for most of us.
That line that represents a circumference is taking on dimensions very fast now. At first we thought it was just a length. Then we saw that it required two dimensions. Now we can see that it is an acceleration. What next?
We’ve gotten to the depths of his kookiness here. In his world, a curve drawn on a graph isn’t something that can represent an acceleration: a curve is an acceleration. In his reasoning (in so far as I can follow it), this is because a drawn curve must have units (it’s drawn, so it’s real and has units!); and since the units that he chooses to apply to it look like an acceleration, then the curve is an acceleration. Alternatively, if you’re drawing a curve, in order for the drawn curve to be a curve and not a line, your hand must be accelerated. The curve is that acceleration.
Once again, it’s pretty hard to know just where to begin with how wrong this is. A curve can be a representation of a physical phenomena; it can describe a value with units. But a curve, in math, is not the physical phemonenon that it could represent. The process of drawing and the thing drawn are, to Mathis, the same thing. In Mathis’s world, a circle drawn with a compass, and a circle drawn by tracing another circle are fundamentally different, regardless of the fact that they’re indistinguishable.
This pretty much defeats the entire purpose of mathematics. One more quote of his, just because it’s so perfect:
Now let us return to the geometric circle. All the equations of geometry are created by assuming that time is not a factor. You can’t really just ignore time, so what the geometry does is assume that all underlying time intervals are equal. What does that mean, specifically? Well, it must mean that all the lines are understood to have been drawn with the same velocity. We can ignore the velocity since we define it as equivalent. What does that mean?
It means that the radius is a velocity itself.
Mathis is remarkably long-winded; the stuff I’ve shown you so far gives you the gist. He just keeps on with the same basic nonsense, building it up more and more. The circumference of a circle, according to Mathis, isn’t a distance. Because, you see, a distance is one-dimensional – but the circumference of a circle curves, so that it’s two-dimensional (Note that that’s his wording, not mine; in another typical example of his confusion, he doesn’t distinguish between the edge of the circle, and the circumference. To him, the curve, the distance, and the process of drawing it are all the same thing.)
All of his stuff really, ultimately, comes down to one basic problem: he is absolutely unable to distinguish between the act of drawing something, and the nature of the thing that he drew.
So, for example, he goes on at great length about how calculus is all wrong. The reasoning comes down to the fact that he doesn’t believe in mathematical points, because you can’t draw them. But calculus is based ultimately, on the concept of infinitely small points. You the derivative of a curve by finding the slope of that curve at a point. But if you draw a point, it’s not infinitely small. It’s got a finite size; to be able to draw it, it’s got to have a finite size. Therefore, there’s no such thing as a point, and if there are no points, there’s no calculus. (He does go on about reformulating calculus so that it doesn’t require points.)
So where does the
You need to go through a whole lot of craziness to actually get to it, but it’s more of the same. The root concept is that there is no distinction between a circle, and the process of drawing a circle. The radius of a circle isn’t a length, it’s a velocity. (Or
a distance. Sometimes it’s one, sometimes the other. But we won’t worry about that; consistently is the hobgoblin of a small mind!)
So what’s
Well… that’s a bit tricky, because he redefines terms like bloody crazy.
But, basically…
He’s rewritten the equations of orbital dynamics as 
Now… you can rewrite that equation as 
See, it can’t be a straightforward acceleration… because, in his universe, an acceleration is a force with a direction. That’s fine. But… there’s no such thing as a point. And in an orbit, because it’s going in a circle, the direction of the centripetal force changes from instant to instant. But there’s no such thing as an instant, because an instant is a point in time. So there’s no such thing as an orbital acceleration, because the acceleration changes from instant to instant. So, therefore, it’s not an acceleration, but rather a velocity. (No, it doesn’t make sense. Don’t worry.)
But… By his reasoning above, we’ve shown that the circumference of a circle is measured in units of velocity squared. So you can’t talk about 
But if you continue with his reasoning… Well, let’s not really continue with his reasoning – he takes his time, and frankly, this is giving me a headache. The gist of it is: 
I’ll close with his summary of what he’s “discovered” in this mess.
We have discovered several important things.
- Pi is a centripetal acceleration and has the dimensions of acceleration.
- The circumference of any circle has the dimensions
, if written out in full.
- If the radius is treated as a distance, then the circumference has the dimensions
- Pi is not applicable to orbits or most other physical circles, since the tangential velocity is not equal to the radial velocity. There is no pi in the sky.
- In orbits and all other circular motion
. Something may equal
, but it isn’t a velocity.
- There is no such thing as orbital velocity. There is only tangential velocity. The curve described by an orbit is not a distance, nor is it a velocity. It has the dimensions
Insanity. Sheer, utter, insanity. This is the kind of rubbish that makes me want to poke my own eyes out, just so I don’t need to look at any more of it.
Not say that he isn’t totally wrong, but I didn’t find this one as crazy as the last crank you came up with. Guess I’m just a sucker for people with the time-cube approach to describing the universe.
He’s more coherent than the last guy… But less crazy? I don’t think so.
The last guy, Mehdinia, was just arguing that there’s a calculation error in the standard computation of
. This one is claiming that the circumference of a circle isn’t a length, but rather some strange entity measured in units of velocity squared!
The difference between the two of them is that the other pi guy was completely delusional to the point of being unable to string together words in meaningful ways. He couldn’t maintain a coherent thought long enough to finish a sentence.
Mathis, on the other hand, writes books full of this stuff. And on a linguistic/grammatical/structural level, it all makes sense. But on a semantic level, when you actually look at what he’s saying, it makes far less sense than Mehdinia. And Mehdinia limits his craziness to just
. But for Mathis, this whole 
this is pretty much just a tiny digression from his main thrust. Mathis believes that he’s a revolutionary genius the likes of which the world has never seen before. According to him, he’s smarter than Newton, Einstein, Leibnitz, Bohr, Gödel, Euclid, and Descartes put together.
That kind of grandiosity puts him way, way beyond the likes of Mehdinia.
I’m surprised at how much of that I just read
Bah. I like this proof that pi is 4 better: http://28.media.tumblr.com/tumblr_lbxrvcK4pk1qbylvso1_400.png
I still don’t really understand why that doesn’t work.
For the same reason smaller and smaller steps never become a straight line. Limits are tricky and have to be used carefully.
That “proof” cracks me up. I love stuff like that. It does take a few moments to figure out why it’s wrong.
Basically, to use the obviously flawed terms in that “proof”, if at step infinity you arrive at a circle, then at step infiniti – 1 you have a really kinky roundish shape.
That really kinky roundish shape is of length 4. Pull out an iron and flatten out the kinks and what you’re left with is π.
Visually that’s pretty neat.
lily, at infinity the outer shape is a fractal rather than a circle.
By doing the shown division to infinity, you end up with this:
/
/
(the same square, rotated)
not the circle.
This is not true. In order for the square to rotate, the line needs to extend in the direction of 45 degrees which clearly does not happen.
What is intrguing is that the area of the reduced square is getting smaller and smaller while the perimeter is remaining the same. At infinity the area is likely to have come close to that of the circle while perimeter is still remaining the same.
Not focussing on the area is a problem with all these explanations that keep calling the final shape a fractal or bumpy. It does not matter, a figure which is clearly larger in area will approach the area of the circle but not in perimeter? There clearly is a problem the explanations are not good enough.
The area of the square-cornered figure seems to approximate the area of a circle as you let the size of the squares approach zero, which naturally makes you assume that the circumference would as well, but it doesn’t.
In order for the circumference to converge on the circumference of a circle, you would expect to see the error (or difference between the two) gradually diminish towards zero. But the error is constant, because the circumference of the square-cornered shape never changes. Or in other words, no matter how small you make the squares, you could always zoom in and see an arc of the circle being replaced by two right-angled line segments that take a longer path to reach the two endpoints of the arc, i.e. an overestimation of the circumference that is proportionally constant at any scale.
You could probably play this game to claim that Pi was any value just by choosing the right shape.
Better even, you could choose the shapes in such a way as to make the Pi infinite. The procedure is one of the classics to build self-affine fractals.
But this one is actually clever, compared to the kookiness in the title post. And the crazy old man’s head in the picture always makes me laugh.
I also like this one:
http://i.imgur.com/IKFiu.jpg
Actually, it has to be added that the original procedure Archimedes applied was to provide an upper bound as well as a lower bound to the circumference of the circle. By showing that both bounds converge to pi, he would then be able to use the construction as an approximation to pi.
In that sense, the picture is only providing an upper bound, which is correct. It’s just not a sequence of bounds that converges to pi. So it’s not an optimal choice.
That is silly argument in my opinion. We use and accept this technique for area under a curve by taking a very small delta and summing the areas of rectangles of very small width as if they are rectangles ignoring the curvature which is the basis for integral calculus.
while i am still trying to explain out the reason why this fails, the construction of riemann integrals is done in the same way as mentioned above, the limiting upper and lower bounds have to match for the integral to exist. I do agree that the construction is just that, a construction. But the area under a curve paradigm actually borrows ideas from this construction.
It might help to see a simpler case where it fails. Cut a unit square in half along the diagonal, and apply the same procedure to one of the triangles. The sides stay at length 2, even as they get closer and closer to the diagonal. So this would seem to indicate that the diagonal has length 2 as well, but (as others have noted) the error stays constant. It’s even easier to see in this case.
I like this explanation that John gave. I’m going to restate it just so that it’s clear in my head. 🙂
Let L be the length of the main diagonal of your unit square. We want to show that L=2.
You perform this ‘subdivision’ process along the main diagonal n times. You would have replaced one large triangle with n “tiny” triangles that zig zag their way up the main diagonal.
Take one of these tiny triangles. It would have two sides of length 1/n, and the side shared with the main diagonal would have length L/n.
The error in this one tiny triangle would be 1/n + 1/n – L/n = 2/n – L/n. As you increase n, it looks like the error shrinks. But that is just the error in one tiny triangle.
You have n of these tiny triangles that sit on the main diagonal, so when you add the total error over all of the tiny triangles it would be:
Total Error = n*(2/n – L/n) = 2-L.
But that’s the error you had when you started, and since the total error does not depend on n it is constant. The only way to use this proof is to show that the total error goes to zero. But the only way to do that is to assume that it is true.
Mathis has a way around that, he claims the diagonal can’t be approximated, because “in some sense it is the length”, whatever that means.
The fact that you could use this to show all monotonic curves connecting the same two points have equal length also doesn’t bother him. He claims you can only use this method for a circle because it has constant curvature, so both pieces of the right angled approximation have equal length.
The strange thing is that he knows what kind of (incorrect/crazy) results can be arrived at using his various ideas, but then explains them all away with the greatest displays of hand-wavery I’ve ever seen.
The way I see it, pi is 4 if you use a taxicab metric where you define the distance d = | dx – dy | rather than the familiar d = sqrt(dx^2 + dy^2).
=] thanks everyone
That is indeed clever, and challenging to spot the flaws.
The observation that we are constructing a series of shapes with constant perimeter but shrinking area is what helps me visualize it.
What you have constructed when you let n approach infinity is a shape of infinite bumpiness, which traces the perimeter of a circle but adds in an extra ratio of 4/Pi in “bumps”. Kinda neat actually.
Heh, makes me wonder if you could make a similar construction with shapes other than squares…
I have come up with a related construction that “proves” Pi = 2.
Start with any equilateral N-gon (use a square for simplicity, but you get the same result with any N-gon). Let each side have a length of 1, so the total perimeter is N.
On each side, bisect it and construct one semi-circle bulging out, and another semi-circle bulging in. This creates a “wiggly” shape that approximates the N-gon. Each semi-circle has a diameter of 1/2, so it has a circumference of Pi/4 (remember, it is a semi-circle, not a full circle). There will be 2*N semi-circles, so the perimeter of the “wiggly” shape is 2*N*Pi/4 = N*Pi/2.
Next, divide each side of the N-gon into four equal segment, and construct semi-circles on each segment, alternating between bulging in and bulging out. Now we have an even “wigglier” shape, with a perimeter of 4*N*Pi/8 = N*Pi/2.
Continue in this fashion to construct shapes with more and more (yet smaller and smaller) wiggles. The area will converge to the same as the N-gon, but the perimeter of the “wiggly” shape remains constant at N*Pi/2.
As the number of “wiggles” approaches infinity, the perimeters must converge (well actually they don’t, but that’s the trick, ain’t it?) and therefore N = N * Pi/2, which translates to Pi = 2. Again, this works with any equilateral N-gon.
The corner cutting method provides a perfectly valid UPPER limit on the value of Pi, but to prove that it converges to a normal circle, you’d have to generate a lower limit that also converges to four. Good luck with that. 🙂
The obvious conclusion is that it converges to a jagged circle, which is different from a smooth circle.
I love fake proofs, because they force you to really understand the fundamentals of what’s going on. It’s easy to look at a valid proof and go “yep; yep; yep”. It’s much more enlightening to be forced to find flaws or misapplications of principles.
The version I heard is that the arc length of a (assumed smooth for pedagogical reason) curve (x(t ), y(t ) ) in R^2, given by integration of sqrt( x’ ^2 (t ) + y’ ^2 (t ) ) (note it is x’, the derivative of x with respect to t), is not a continuous functional of the curve (see PS1). That is, if we have a sequence of curve C_n = ( x_n(t ), y_n(t ) ) “converging” to C* = ( x*(t), y*(t ) ), the arc length of C_n does not necessarily converge to that of C*. And that’s because differentiation (appearing in the formula for arc length above), unlike other operations (such as integration), is not a continuous functional.
PS1: Even if we are talking about continuous functional in the stronger sense of “sup norm” // “uniform norm”. For the layperson, a sup norm means roughly the largest distance between the two curve. The effect is that instead of merely requiring the curves to “converge” on each point, possibly at their own rate, there is some kind of “control” on the rate to be at least this fast.
PS2: And this explanation, while being technically satisfying to me, is unfortunately advanced (real and functional analysis just to deal with a circle?! )
I like math. I like it enough to be almost done with a (useless) social science degree and to decide to switch to a math major, starting with trig to get myself back up to speed, and essentially going through all the basics to get my math degree.
That, right there? If that was supposed to have made sense, I would be dropping this math major idea right now.
As it stands, I’m going to go enjoy doing my homework and being way less brain-hurty.
Well, I guess the π is a lie, then…
“There is no pi in the sky.” Was the whole thing a lead up to this pun?
Pi represents C:2r in flat spaces; for curved spaces, that ratio may be different. For example, if you are measuring circumference vs diameter while stuck on a spherically curved space, C/2r=2 because your measured diameter consists of you walking halfway around the globe in a direction tangent to the one in which you measured circumference. One can even imagine spaces where the ratio is not constant, but changes based on the direction you use to “walk” the diameter. Imagine a cylindrical space with no caps: in such a space, you could draw a number of circles. But if you draw one particular circle by walking the circumference of your space, you are in trouble. If you align yourself perpendicular with the circle and start walking to measure the diameter of your circle, you will never reach the other side. 🙂
But even considering such cases it is stupid to say pi ‘changes.’ The ratio C:2r changes. Pi remains a useful mathematical constant no matter what space you’re considering.
When I first started reading, that’s what I thought his argument was going to be: “Euclid was wrong because not all spaces are Euclidean!” (derp) Which doesn’t make Euclid wrong; Euclidean spaces are still obviously very useful. In fact, not having been a math major, I daresay that I really don’t have a strong mathematical understanding of any other space (though I can visualize how all sorts of Euclidean assumptions are violated on the surface of a sphere).
But it’s much crazier than that, of course.
On the right surface you can have the radius of a circle greater than the circumference. Amazed me first time I realized that. For example consider the surface z = 10(x^2 + y^2), and the circle on it determined by z = 10.
The circumference of the circle is 2*Pi =~ 6.2832, but the radius is approximately 10.1047.
I know it’s simple, but I thought it was amazing the first time I thought about geometry on anything other than the plane.
Haha, I didn’t know about this site, its great! I’m glad you guys took a look at Mathis
work, some of the errors are just unbelievable.
For those of you who are interested I had a look at Mathis paper on kinetic energy
and wrote what I think is completely wrong about it here in this thread
http://www.thunderbolts.info/forum/phpBB3/viewtopic.php?f=8&t=2582&p=41885#p41885
and I’ve been talking to Mathis supporters trying to get them to defend the
arguments in the paper. Needless to say all I got was dishonesty and sidetracking
but if you think I’ve made any errors please let me know, I don’t think I have but
I’m always worried :p
There’s another analysis of Mathis mistakes on π = 4 here:
http://mathisdermaler.wordpress.com/2010/11/15/a-reply-to-%E2%80%9Cthe-extinction-of-pi-the-short-version%E2%80%9D/
Looking at a few things in my office, I find at least four manufacturered objects with circles in them:
– the top opening of the ceramic mug out of which I am drinking hot chocolate (mmm hot chocolate)
– the lock on the nearby overhead cubicle cabinet
– the vent cap on the end of the HVAC air vent that blows cold air on me in the summer
– the box supporting (and holding the wire for) the lamp over my colleague’s desk
Every one of the circle objects described above was manufactured with the working assumption that π is, for lack of a better term, the consensus value.
Surely these objects would not be circles if it was instead a different value?
Or does Mathis have an answer for that objection?
He has a sort of answer for it… It’s based on a rather arrogant and pig-ignorant hand-wave about engineering.
See, engineers don’t need to actually understand what they’re doing. They just do whatever-the-fuck works. So when things don’t work the way they want them to, they just add slop factors for error correction until they work. So, hidden in the manufacturing process for those round parts (and in the computation process for satellites, airplane routes, paving requirements for traffic circles, etc.) is a slop factor which, if extracted, would prove that the computation is really using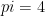
.
Don’t let the fact that slop-factors like that don’t exist worry you. Nor should you worry about the fact that slop-factors like that wouldn’t work. Because the only reason that you believe that those things don’t work is because you don’t understand that the circumference is a velocity squared.
See how easy it is to argue when you’re a crackpot?
I don’t suppose that slop factor would be something like 3.14/4, then, would it?
Beware! You’re going to get an influx. I commented on his insanity here, and then ended up in an argument (which I just ended, because it was rather stupid) about his derivative insanity.
I’m impressed at how he doesn’t seem to think there’s anything inconsistent about this. It must take a lot of work to convince one’s self that such a thing is correct.
This is because time is dimensionless…
Mathis has offered up another explanation for his Pi = 4, he uses the “stair-step” method mentioned above. Nice little geometric proof that proved nothing but his fundamental lack of understanding. Check out “The Extinction of Pi – The Short Version” at http://milesmathis.com/pi3.html.
I will note that in the long version he states something to the effect that Pi=4 only applies in a kinematic situation, but for “measureing your waistline” (his words, I assume he means plane geometry) we can keep the current value. Then in the short version he tries to use plane geometry and a limit to prove Pi=4.
There are a couple guys who started a blog with the purpose of debunking Mathis arguements. I decided to contribute a couple articles to their blog and do my little bit to help ensure no young mind is led down he path to crankdom. Their blog can be found at http://mathisdermaler.wordpress.com , and my first submission at:
http://mathisdermaler.wordpress.com/2010/11/15/a-reply-to-%E2%80%9Cthe-extinction-of-pi-the-short-version%E2%80%9D/.
Mathis is so inconsistent that it can be difficult to argue against him. He says a radius is a velocity, so you demonstrate this makes no sense. Not to worry he says, you misunderstood, the radius is an acceleration. Again you demonstrate this makes no sense. Not to worry he says, the velocity of the radius = velocity of the circumference (apparently this is a postulate in his little world). Again you demonstrate this makes no sense. Not to worry he says, since in the history of man he is the only one to have the mental ability to truly understand these problems it is not surprising that your simple mind cannot comprehend the complexity of his genius. Go home and play with some lego.
Unbelievable, absolutely gob-smacking Unbelievable. I’ve had a quick look at Mathis’ work and yes it’s gobbledegook (As if I even needed to say that). However, I did wonder if he is cynically bating established science and knows that he is simply pushing nonsense using (in his own words) “for the most part.. high school level algebra”. But although he is a dissenter and doesn’t believe established science he really does believe in himself. As is so often the case, the ego is the key. You might win the argument but you won’t win him – his ego is so big that you may as well attack a death star with a paper dart. In studying Mathis works you are not so much studying science but studying the world of Mathis, which of course is what the cosmos is all about as far as he’s concerned.
Trouble is, Marhis is likely to pick up disaffected anti-scientific establishment people out there for whom modern science has gone right over the horizon and for whom the use of elementary algebra will appeal. It is Mathis’s ironic opinion that: “Simple math ….. cannot be used as ballast, as misdirection, or as obfuscation.” (!!!)
Mathis is a terrible time waster and attention seeker. Just look at this self portrait he appropriately calls “Me”. http://mileswmathis.com/me.html Is he in love with himself? And here’s the man himself : http://www.youtube.com/watch?v=I6qI3nH9IOE&feature=related.
I agree we are off the crankometer scale here;. I’ve never seen the like of it. Nothing is untouched; even some “Cantor crankery” in there to boot. However, to be fair I couldn’t find anything on Mathis website about perpetual motion. But perhaps I haven’t looked hard enough.
Point well taken. The self portrait says it all. I will also add that somewhere in his ramblings he says his mother was a professor of mathematics and has told him that he is plain wrong. If it is true that his mother is a professor of mathematics and he still refuses to accept even the posibility he could be wrong, then you are absolutely right. He is not writing this crap in an attempt to add to science, it’s pure mental masturbation.
Why is it all cranks share the idea that some simple algebra, a splash of math/governemt/corporate conspiracy theory, and a little folky wisdom can somehow Voltron-together and tackle even the most difficult of problems?
It does appear he can paint and sculpt though. Perhaps if we asked him to sculpt a hyperbolic surface he could Voltron-together his artistic skill and mathematical genius to produce something kinda cool… maybe
It’s surprising given his artistic side that he has not “investigated” projective geometry.
If you can’t tell… this guy seems to have really got under my skin. It’s not the questioning of current methods that bothers me, it’s his arrogant dismisal of everyone who ever had a mathematical thought before him.
…getting under people’s skin is the name of the game! Where Mathis genius lies is clearly not in mathematics and physics, but in being a phenomenon; simply getting noticed by being Miles Mathis. If being a “crank” is a kind of perverse talent, then I think he’s got bags of it; far more talent than the other crackpots Mark has brought to our attention. If I were a reality show producer I might be interested in Mathis. As for the rest of us mortals we plod along playing by all the rules and no one gives a damn. The irony of it all is that we are simply helping him on his way by making him a discussion point. Basically he’s riding on our backs. Too right he gets under our skin!
There’s a new paper by Mathis on his site in which he actually says the following:
“The history of science is a history of great individual thinkers, of Archimedes and Leonardo and Galileo and Kepler and Newton and Einstein. It is not a history of committees and peer groups. Galileo did not succeed by a vote. Newton did nothing with the authority of a majority. Just the reverse. All these great people did what they did against the majorities of their times. You only have to study their lives to see that, in science and other hierarchies,
the majority is always wrong.
In both society and in science, the majority is always staunchly arrayed against anything new. They always were and they still are.
That alone destroys the rationale of peer review, since a person with a new and better idea has no peers. ”
http://milesmathis.com/1920.html
:p
Honestly, this is crazy stuff. I can only guess as to what motivated him to write
this newest paper 😉 Also, another nice quote:
“He or she has the idea and no one else has it. And, in most cases, the new and better idea is not immediately comprehensible to those who did not have it:”
So yeah, you’re all wrong about this pi business because these ideas are so new
they are not immediately comprehensible, makes sEnsE, don’t it?
Apparently to Mathis there is no science nowadays because we’ve democratized and
publish peer reviewed papers. By this logic what happened in CERN the other day,
creating an anti-hydrogen antimatter particle & sustaining it for an extended period of time, is not actually science. I feel so edumacated!!!
He is bracing himself in this paper, he is saying that we are out to get him no matter
what, if he has a few ideas wrong (such as those we’ve all shown over the past week) then he’s a crackpot, if he has everything wrong, hes a crackpot, it’s win win for us according to Mathis because we are like that, we’re democramatized cronies…
It’s nice to know he can not only correct my ‘gloriously negligent textbook’ he also
knows how I think and feel about his material in a deeper way than I apparently do,
I’m glad he is able to speak for me too because it’s his clarity & depth that will soar like a lonely bird when all is said & done to fly North through the annals of history,
I’d hate to have missed that clarity & I am aware of it now from reading this latest of his scientific papers, hopefully you will too…
“will see it too…”
Here’s a little quote from one of his papers, it was too fantastic not to share:
“Now let us look at complex numbers. Curved geometry is often used in conjunction with complex numbers. Well, complex numbers can also be stretchy. A complex number is in the form x + yi, where i is the imaginary number √-1. Now, like the number 1, this number should be firm. It should not vary. The √-1 should always be the √-1, and it should not change size or shift value willy-nilly. But in modern manipulations, i is not always used as a firm value. No, it is sometimes used more like an infinitesimal. It can change size depending on the needs of the mathematician. In other words, it is a fudge factor, hidden by a letter that confuses almost everyone. Many people seem to think that i is a variable, since it is dressed as a variable and sits next to variables. But it is not a variable. It should not vary. Treating i as a variable is like treating the number 5 as a variable. I hope it is clear that the number 5 should NOT be a variable in any possible math, since in any problem the number five should have a firm size.
Complex numbers have an even more important role than supplying this room to move. Complex numbers were invented to hide something. What are they hiding? Let us see.
Wikipedia, the ultimate and nearly perfect mouthpiece of institutional propaganda, defines the absolute value of the complex number in this way:
Algebraically, if z = x + yi
Then |z| = √(x^2 + y^2)
Surely someone besides me has noticed a problem there. If i is a constant, there is no way to make that true. That equality can work if and only if i is a variable. But i is not a variable.
Let x = 1 and y = 2
i = .618
Let x = 2 and y = 3
i = .535
Let x = 3 and y = 4
i = .5”
& after that passage is my personal favourite:
“The fact that i equals anything is a major axiomatic problem, since it can’t equal anything but √-1, and √-1 is nothing. The √-1 is like a unicorn or a fairy. We should put a picture of a griffon in the equation instead of a cursive character. Or how about a clover as our lucky charm here? “
“√-1 is nothing. The √-1 is like a unicorn or a fairy.”
I forget where, but I read one explanation of what i represents as: consider a typical cartesian coordinate system. Multiplying by -1 is analogous to reversing direction or a 180 degree rotation (transposition?). Multiplying by i thus represents a 90 degree rotation.
I think that was here – that’s the way that I like to explain complex numbers. Complex numbers are numbers where you’ve added a dimension, so that they’re two dimensional.
Personally, I actually think that there should be an “r” – because in a complex number, when we say “x + yi”, what we really mean is “x”
in one dimension, and “y” in the other. i is, in some sense, a counterpart to 1 in the second dimension. Except that when we say “1”, what we really mean is pure magnitude. Just like we use an “i” to denote “magnitude in the i dimension”, I’d prefer the notation to use something to denote “magnitude in the r dimension”.
But, anyway… In practice, many of the applications of complex numbers do involve rotations; and multiplication by “i” does, basically, mean rotation in the complex plane.
I need to take this back to the my prof from my first complex analysis course. At the end of some question I wrote ln(z) + 2*kP*iwhen I hsould have wrote ln(z) + 2*k*i*Pi, lost half a mark. That bastard knew it was a fudge factor yet still robbed me of my half mark.
On the amazon.com review for Mathis’ book you will find the last line of one review reads “To wrap it up: IMHO the most lucid scientific mind I’ve ever seen, now in paperback. A modern day polymath on its way to history as a new Leonardo.” – Steven Oostdijk
If you were to look around various math & physics blogs where ever you find an argument against Mathis you will inevitably find “Steven Oostdijk” there to support Mathis. I suspect Oostdijk IS Mathis. Although I can’t prove it, there is pretty compelling evidence.
The best evidence that to me trumps all other forms of evidence is
Mathis comment on the paypal button:
“If this paper was useful to you in any way, please consider donating a dollar (or more) to the SAVE THE ARTISTS FOUNDATION. This will allow me to continue writing these “unpublishable” things. Don’t be confused by paying Melisa Smith–that is just one of my many noms de plume. If you are a Paypal user, there is no fee; so it might be worth your while to become one. Otherwise they will rob us 33 cents for each transaction. ”
Basically he is letting us know he isn’t one to shy away from adopting a nom de plume or 2 online. If he’s willing to actually receive money in the
name of a woman I see no reason to think he would not fake some story
under the guise of Oostdijk as being an amateur supporter who is in contact with him to do experiments. Also, I am almost sure that in
one set of comments Oostdijk says he’s never contacted Mathis & has
no affiliation with him while in another he says he’s in contact with him
organizing experiments. I’d have to look to find them to be 100% sure I’m right, & I could be wrong, but even based on the linguistic similarities, the style of response, the use of certain words etc… I am
not fooled. But then again, these could be just similar cognitive traits for
those who delve in these matters :p Reading some of the comments sections in posts on this site I do wonder :p
I dont know, I can’t find the comment where he said he had no
affiliaition with him, I take it back as I can’t prove it & probably have confused things. If Oostdijk is him or not matters not, it’s
only of interest because so many signs point to this & it seems
like such a charlatan thing to do to hide like that while leaving
breadcrumbs & lie about it. Whatever the answer it doesn’t
change any of the errors he’s committed in his papers. Im glad the
insanely insulting rhetoric constantly employed in his “scientific”
papers is coming back to bite him on the ass because it’s so
horrendous & spiteful, the second he began those insults he
gave everyone every reason to use the slightest mistake on his
part as a chisel against this outward persona of his. We might see
more meat in his papers & less insulting self-congratulatory
rhetoric seeing as he is extremely capable of ridiculously childish errors as well, nobody is really as immortal as the
illusion a portrait gives off. Would everyone have reacted
the way they did had he not been so ridiculous with the snide
commentary and grandiose self-perception? Are we all really
just slaves to power and nothing but democratized cogs in
the wheel? Are we just brainwashed into ignoring the valiant
efforts of the lone ranger doing what we all would like to but
can’t for fear that the democratized majority, the herd, the
cattle, the drones, the [insert misanthropic comment here]
will disapprove of us? Reading Mathis it’s pre-destined that
I am apparently. In fact, reading Mathis it’s predestined that
these people even exist! What a beautiful view of humanity…
I have definitely seen the the post somehwere where Oostdijk claims to be setting up experiments to do with Mathis. I’m pretty sure I also saw another comment where he claimed they did not know each other. You’re right, are just too damn many similarities. Another damnig piece of evidence is Oostdijk’s unwavernig support for anything Mathis has ever written. Even in the face of proof Oosdijk will not even consider the possibility he’s wrong. He also claims to understand what he means with his whole “velocity of radius = velocity of circumference” crap. It’s total crap, if anyone with even the slightest ability for analytical thinking read that they would immediately see it made no sense, so I propose that either Oosdijk lies when he says he gets it, has no capcacity for independant thought, or he is in fact Mathis.
He also pops up anywhere that Mathis’ vanity published “physics book” can be bought online and offers nothing but praise for “the new leonardo”.
I’ve noticed that neither Mathis nor Oosdijk are willing to use a Mathis theory to make a single prediction. They have both been asked to propose an experiement where the Mathis model will make a better prediction than the standard model, but these requests are ignored.
I think the whole Oostdijk persona is Mathis’ attempt to offer some perverted sense of peer review.
My personal favourite is the claim that the definition of the derivative is wrong. h->0 is impossible, since 1 is the unit or “smallest number” h->0 maks no sense. Also instead of d/dx x^n = nx^(n-1), the *true* method of finding the derivative is to keep taking the “fundamental acceleration” (derivative I think) until you reach a linear function, then somehow handwave that into a derivative. When I asked them how to deal with x^3/2 or x^(-2) there was nothing but silence.
But alas, since I do “nothing but parrot my textbooks” what do I know.
I think my favorite quote is
I understand that he thinks that the cardinality of the Reals is equal to the cardinality of the Rationals, but does he think that there are only finitely many rationals in the set [0,1)?
sorry, the above should read:
… I wrote ln(z) + 2*k*Pi when I should have written 2*k*i*Pi…
I’ll join in the foray here for fun. He writes in his book recommend by someone works for *NASA*
“Any logical system is set up to avoid the necessity of proving axioms or postulates.”
Well, usually axiomizations of propositional calculi come as theorems in other formal systems. E. G. for the axiom set {CqCpq, CCpCqrCCpqCpr, CCNqNpCpq} CCpqCCqrCpr, CCCNppp, CpCNpq are theorems and vice versa also.
“A logical system is a closed system in which axioms and operations define the total extent of any consistency (or truth).”
No, the axioms can get verified outside the system. E. G. truth table can verify the above axiom sets as valid. Or the axioms of one set can get used to derive the others.
“The system as a whole therefore requires no proof, and any reference to anything outside the system in illogical in itself.”
No, one could consider CqCpq as a sole axiom for propositional calculus. We can’t derive everything in propositional calculus with just that axiom.
“Deductions require no proof beyond their own postulates.”
Well, if that is so, then I could write a formal proof like this:
1 CqCpq
2 CCpCqrCCpqCpr
3 CCNqNpCpq
———————
4 CpCNpq
5 CCCNppp
6 CCpqCCqrCpq
Or like this:
1 CqCpq
2 CCpCqrCCpqCpr
3 CCNqNpCpq
———————
4 Cpp
(note, as arguments one can consider those as valid, but they are NOT formal proofs, because the question of getting from the axioms to the theorem(s) isn’t answered in the slightest detail in the text).
Oh wait, I have to use a *rule of inference* such as the rule of substitution or the rule of detachment which isn’t in the set of postulates {CqCpq, CCpCqrCCpqCpr, CCNqNpCpq}. This is true for both natural deduction and other types of systems.
“Only inductions or inferences require outside proof. That is to say, connecting one logical system with another requires proof.”
But then you’re proving axioms! You said you couldn’t do that Mathis, and now you’ve claimed that connecting logical systems requires proof, which implies that axioms get proven.
“This proof is an additional set of axioms or operations that are also accepted without proof.”
No, because you prove the axioms just from a distinct set of axioms.
“But this is not what proof means in logic. According to the rules of logic, a proof must have a starting point. This starting point is an axiom or postulate. This postulate must be logically unprovable. To try to prove it is to misunderstand logic itself.”
Ah, but not only can it get done Mathis, it *has* gotten done, *several times*. So, in logic it *is not* logically unprovable. On top of this, it at least seems that hardly anyone starts with a single axiom or postulate. Of course, such can and does get done. But, not enough to warrant such a statement.
Alright, hopefully it comes as clear that Mathis is illogical not only figuratively but literally.
proof, schmoof. Why not do it legislatively. Indiana tried; I believe the value was 3.2. Now with the (WARNING POLITICAL OPINION AHEAD) Tea Party on the upsurge, another attempt may succeed; and with the proper application of states rights, it could have different values in different states.
The days of crazyness like legsitlating the value of pi are behind us, I hope, but there are still serious concerns about outside influence on the scientific community. I suppose an argument could be made for some government oversight of some science, for both moral and practical reasons. Studying genetics is all wonderful and everything, but the applications of the research require some moral guidelines. Perhaps any research that could be easily weaponized might need some oversight. There are people more qualified than I to debate just how much if any oversight is appropriate in these cases.
I am however of the opinion that government has no business in matters of mathematics. I know there has been discussion on patent and copywrite relating to numbers, specifically in relation to encryption keys and deCSS. You know the jerkoffs responsible for conservepedia, the Schaflys, one of them even sucessfully patented two primes numbers. I believe the patent only applies to using the two primes as part of some algorithm, perhaps cryptographic, I’m not sure. Whatever it applies to, patenting numbers is absurd. Suppose this or that number was essential to a proof, does the author of the proof need permission to use it, or would royalties become an issue?
I’m all for protection of intellectual property and the free market, but a line needs to be drawn somewhere. How can someone own a number? Admittedly I know absolutely nothing about genetics or related fields, but the notion of patenting part of the human genome or sequences of human DNA is outrageous. Perhaps I’m speaking out of ingorance and don’t really know the facts, but the idea that some company can exert some any control over what can be done to this or that gene in me is discusting. Sure, patent a medicine, but how the hell can a gene be patented so they are the only ones who can develop anything that relates to it? If my admittedly little understanding of the issue is incorrect, then I’m wrong, what can I say? If I undertand the basic ideas correctly, I am outraged.
Those are my two cents an an issue that really rubs me the wrong way. The government already wields considerable influence on the direction of research as they are the largest single funder of universities. Government and corporate influence on the direction of research is inevitable, but any further influence should be firmly resisted. Don’t get me wrong, I’m not some anti-corporate anti-government nut, I realize they are both needed for funding, I just believe certain things should be held sacred and not be subject to patent or copywrite claims.
I got a little off topic…. forgot to mention
Mathis is a kook! Check out:
http://www.hyperdeath.co.uk/kook/analyse.php?name=Miles+Mathis&data=keyaydywvddavrffhttp://www.hyperdeath.co.uk/kook/analyse.php?name=Miles+Mathis&data=keyaydywvddavrff
“The days of crazyness like legsitlating the value of pi are behind us, I hope…”
No, by definition they are still ahead of us, given that no such bill ever passed.
The bill in question was submitted by a crackpot, but postponed indefinitely once a concerned citizen told the legislators they were being baffled by bulls**t. This is not too different than what happens today – legislators rarely or never read entire bills before voting on them; it generally takes an outside force to get them to reconsider a bill.
This is in some respects an example of the success of the democratic system – not an example of its failure – since in this case the legislative process acted as a break on a crazy idea.
…oops, that should be “brake”
I’m suddenly reminded of the following: If you take the old definition of a meter, the length a pendulum must have in order that it has a half-period of one second, then in this system of units, Earth’s gravitational acceleration is g = pi^2 m/s^2 = 9.869… m/s^2.
If one then gets the interpretation of this completely wrong, you could conclude that pi is not dimensionless, and that pi^2 is an acceleration.
Perhaps his reasoning is a bit akin to this?
So, IF, a whole number set {0.1.2.3…} is a code. Every number following the first defining integer in that set must agree with that set. So, 1 dog = 1 dog plus one dog is two dogs. Then, (and this is why many people steer away from Pythagoras and Euclid. Except for you university types who really lean on your degrees to eat ). You cannot have an infinite number, WITCH would be validated by an equation, WITCH began using a set of whole numbers. Why? Because at a measurable spot along a linear definition of that original number set, YOU, the user, made the jump from rational defined parameters to an irrational solution based upon the original whole number set. HOW FUCKING HARD IS THIS TO GET? Seems that the more brainy you become the less you understand. When you include an infinite number into a predefined set of rational numbers then the very physical sense of volume, or finite measurement, for that set is undermined. So, the original set of numbers that YOU used to begin your math is now compromised. You created the paradox. You compromised the set. There is no such a thing as half a dog. Or 1.5 dogs. They are not dogs anymore. They are something else. You have to go back to square one and re-define what it is we are working with. 10 pounds of meat and bone.
So half of your terms make no sense, or are ambiguous in meaning (code? agreeing with a set?). I assume that “validated by an equation” means “reachable by induction”. If you literally mean an equation, please provide one. And all you’ve done here is show that transfinites are not whole numbers. congratulations.
“Physical sense of volume”? “finite measurement”? These are abstracted from the real world, and don’t necessarily represent physical quantities. You started by talking about dogs, and then swapped to volume, and at the end you claim that you were talking about dogs the whole time. The original set is not “compromised”, there are just some numbers that exist outside of the set. You’re right in that 1.5 dogs doesn’t make sense. But that doesn’t mean that 1.5 doesn’t exist as a number, just that you can’t apply it to counting things that exist in discrete whole quantities. Yes, fractions are “something else”. They aren’t whole numbers. But that doesn’t mean that they don’t exist, or that whole numbers are invalid because something other than them exists. Make sense? If anything “compromised the set” it was YOUR assumption that transfinites and fractions were part of the whole number set in the first place.
You may call Miles Mathis all you want and superficially he’s a complete crackpot, obviously. However, on a deeper, more fundamental level it is evident something is bothering him and in a way he has a point. What is bothering him is the overemphasis on mathematics when drawing conclusions in physics let alone the more than infrequent tendency for fudging and outright cheating in some basic derivations in physics. What bothers him, judging from his texts when reading between the lines, is the lack of clear understanding of motion in contemporary physics which calls for reworking of the incomplete Newton’s second law, for instance. The unbridled endowment with physical meaning of the Hilbert space in quantum mechanics also needs to be reconsidered in view of the fact that often eigenvectors that come up after collapsing the wave function have no physical meaning at all and that’s the real problem with quantum mechanics, not its interpretation. Not to speak about one major “theory” infesting physics, which must be abandoned altogether due to its internal contradictions, without any need to be replaced by anything — the way weed needs no replacement when cleared from a wheat field. Nonsense needs removal, not replacement. For some reason which I fail to understand Miles Mathis is barking up the wrong tree in pursuit of correcting the above discrepancies in contemporary physics which have indeed brought it to a dead-end.
Who are the crackpots? There exist faulty proofs in refereed journals. I suggest cleaning up your backyard before picking on one man and his somewhat misguided efforts.
Sure, there are faulty proofs in refereed journals. It’s not being wrong that makes someone a crank. Everyone, no matter how brilliant, is wrong some of the time.
The distinguishing characteristic of a crank is the refusal to accept that there is any possibility that they made a mistake.
Mathis redefines terms in non-sensical and inconsistent ways, and then shouts down anyone who points out that he’s wrong.
isn’t an acceleration, and it’s not 4.
I appreciate the comments concerning Mathis and his bizarre mistakes in science and mathematics. But this faux renaissance man fumbles about in several fields, including art. He is a critic and an artist but he seems to do very little painting and sculpture, preferring instead to criticize other artists with a bitterness which hints at something deeply resentful in his nature. He pretends not to want material success but his virulent rants against gifted artists whose work is eagerly purchased by collectors give the lie to this.
this article sucks. thinking of things in different ways it why we have what we have, know what we know. it’s great to read a fresh approach to thoughts and patterns such as Mathis’. if only the math were developed to properly, lawfully, perfectly measure the amount of worthless ad hominem expressed herein, i do hope that in the grand way of the universe the writers, editors, and proponents of this article learn to face such a measure, such as karma. goodbye scientopia
On a circular flat race track.
Your odometer would show pi but your fuel gauge would indicate 4.
The following is a collection of quips and witticisms [a best of] taken directly from the Miles Mathis web site:
“that solution looks like a fudge”
“fudged from top to bottom”
“a big fudge”
“a blatant fudge”
“a clear fudge”
“a double and triple fudge”
“a flagrant fudge”
“a further fudge”
“a highly successful fudge”
“a horrible fudge”
“a magnificent fudge”
“a major fudge”
“a massive fudge”
“a mathematical fudge”
“a new fudge”
“a non-mechanical fudge”
“a purposeful fudge”
“a triple-decker fudge”
“a virtual fudge”
“embarrassing fudges”
“the biggest cheats and fudges”
“have to be fudged”
“they had to be fudged”
“is a fudge”
“was just a fudge”
“to fudge later”
“fudged and false”
“fudged as well”
“both illegal and a fudge”
“that manipulation was a fudge”
“full of fudges”
“must be fudged”
“this is just one more fudge”
“fudged corrections”
“forced to fudge”
“talk about a fudge”
“to fudge over”
“all the fudges”
“the barycenter fudge”
“the spring tide fudge”
“the standard model fudge”
“repeating a fudge”
“to be fudged”
“based on a fudge”
“pushes and fudges”
“an excuse to fudge”
“correct their fudge”
“fudge the math”
“the moon’s orbit is fudged”
“the whole thing is a fudge”
“room to fudge”
“forced to fudge”
“fudged data”
“that is a fudge”
“there is even more fudge”
“big fudged equations”
“fudge any equation”
“fudge your math”
“another fudge”
“remove all the fudge”
“you fudge your fudge”
“refudging the old fudges”
“just one more fudge”
This is only a partial list; there are many more!
Gee Steve, Miles has sucked you right in.
I lol’d
Hello! I just wanted to ask if you ever have any issues with hackers? My last blog (wordpress) was hacked and I ended up losing many months of hard work due to no back up. Do you have any methods to stop hackers?
Miles Mathis seems like a former high school student who knows that the Earth revolves around the Sun but is out to prove no one has been to the Sun, therefore nobody can prove that the Sun is even real.
What a joke. Why bother writing about him at all? You are just feeding his fire. All of this time and brain power is better spent doing ANYTHING else. Stop wasting your time!
Let’s focus on this; PURPLE IS REALLY GREEN… IF YOU SQUINT YOUR EYES… IN THE DARK… WHILE LOOKING AT SOMETHING GREEN.
Miles Mathis seems to have his own crackpot followers. Take this one for example who is trying to justify the bible with science, and with mathis’ therioes….
http://thesis.christian-physicist.angelfire.com/
See http://infrastruct.blogspot.com/2012/05/why-pi-is-not-4-and-other-mysteries.html
for a proof that there is no contradiction like “pi = 4”.
If you use units where the reduced Planck’s action is set to the unit, i.e.,
, then you get that 
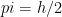. Therefore, if you fix the planck’s constant to some large value like, say 10, you gent 
is such system of units! The key is what we mean by units… 😛 Of course, since Planck’s constant is close to zero, then, in natural units, 
, hehehehehehe Happy Christmas time to everyone out there!
This sounds convincing… it uses mathematical symbols and some physical jargon… I like it! XD π≈0
Pingback: The Pi=4 Theory | Miles Pantload Mathis
“(Or a distance. Sometimes it’s one, sometimes the other. But we won’t worry about that; consistently is the hobgoblin of a small mind!)”
It seems that while you were “squashing bad math and the fools who promote it” you squashed the difference between a noun and adverb. But Computer Scientists can do that, right? Apparently, skills in binary logic mean that you can change the underlying logic of grammar.
You should probably proofread before arrogantly stroking your own ego.
Why put so much effort into ridiculing others?
What does this blog accomplish? What part of you is so threatened that you feel the need to devote so much energy to calling other humans “stupid”.?
What is the difference between you butchering the laws of grammar and this man “butchering” your sacred mathematical calf?
Absolutely right. Also, Newton’s Principia contained a misspelling, so he had absolutely no right to correct Aristotelian physics, and calculus should be wrong.
I didn’t read all of the comments above, but I would like to state that Miles is not saying that pi equals 4, but that in some formula’s where pi is used, it should be 4 instead of pi.
An his prove of pi = 4 is actually a proof that pi is wrongly used to convert from tangent to circulair (in some physic equations. Well, I guess that’s what Miles states at the end of the Original document (long version).
First, imagine a riotous chorus of laughter; people literally rolling on the floor, laughing hysterically. With that image in mind, read the following Mathis quote:
“Quantitatively, this may be THE biggest error in all of math and physics, since every single physical equation with pi in it must now be thrown out and redone. The transform pi must be jettisoned from all of kinematics and dynamics… The circumference equation is now C = 8r = 4d. This means that pi is extinct.” – Miles Mathis
“Insanity. Sheer, utter, insanity. This is the kind of rubbish that makes me want to poke my own eyes out, just so I don’t need to look at any more of it.”
If you are going to “poke your eyes out” (might as well, because you’re not interested in seeing anything new), you might also seal up your mouth from the acrid and bellicose ad hominem spewing forth. Either Mathis is right or he’s wrong, but your opinion expressed in subjective pabulum makes no difference.
I don’t know about the rest of his theories, but it took all of 5 minutes to realize what he was talking about in the calculation of the circumference and dimensional quality and difference between a drawn line and an abstract line, a straight line and a curved line. The shape of the point on the drawn line is different depending on its curvature which is why it can’t be comprised of a single dimension. The abstract circle ignores this entirely, that the inside of the line and the outside are different – there’s no similarity between the one dimensional straight line and the two dimensional curve, which limits the application of π (3.14…) for calculation of the circumference to abstract expressions only.
BTW, the diagram of the stepped circle of increasing granularity works for both area and circumference and is a proper application of limits, and is not a fractal.
Regarding the shape of the points, I predict lining up the wedge segments (the shape of the “points” along the curve), will add up to 4.00000 when lined up point-to-point at the furthest part of the wedge diameter as the width of individual segments approaches zero.
Very nice, except for one very major problem.
Points don’t have a shape.
A point has no length, no width, no volume, no shape, no curvature. By definition, a point has no shape. A point isn’t shaped like a wedge or a line or a square or a circle – because it has no shape.
The moment that you start talking about something with a shape, you’re no longer talking about points. You can “prove” all sorts of things based on the idea that a point has a particular shape, but it’s worthless.
A “Proof” is built on a set of axioms (the built-in facts of the logical system that you’re using), a set of premises ( the know facts about the thing that you want to prove), and the inferences that can be drawn from the axioms and the premises under the inference rules of the logic.
To be meaningful, the axioms and premises used by the proof must be consistent. This isn’t a minor point: if there are any inconsistencies in the axioms, premises, and inference rules, then it’s possible to produce a mechanically valid proof of any statement you want.
For example, in number theory, one of the basic axioms defines how multiplication and multiplicative inverses work. Part of the axiom is that 0 does not have a multiplicative inverse, and that means that you cannot divide by 0. I can easily show you a proof that 2 = 1, by using a division by 0 in the “proof”. Of course two does not equal one! But I can prove that by using an inconsistent premise:
In step 5, I’m dividing by zero, because (x – y) = 0. So implicit in the proof is the idea that I can divide by zero. But one of the axioms says that I can’t. That’s inconsistent, and since I’m building on the inconsistency, false statements are provable.
If you try to prove anything about the shape of a circle or the value of pi by using the “shape” of a point, then you’re introducing an inconsistency. Because in the definition of what a circle is, you rely on the definition of what a point is. In the axioms of geometry, a point is defined as a zero-dimensional thing which cannot have a shape. So when you reason using the shape of a point, you’re reasoning with a system that includes both the fact that a point is zero dimensional and shapeless, and also that a point is two-dimensional in order to have a shape.
It´s well known that pi isn´t 3.14 in curved spaces so I wonder why so much noise, especially 90 years after general relativity. I am more stunned by pi supposedly being an integer number than by any variation of pi in CURVILINEAR motion. Geometric pi doesn´t involve time nor change of direction, a kind of curvature.
Your odometer would show pi but your fuel gauge would indicate 4.
Pingback: Weird Internet Ideas: The Platonic Ideal of Fringe Ideas with a side serve of Voxopedia | Camestros Felapton